judithknott

My EU-flag cushion is finished at last. I’ve spent the last couple of days frantically sewing it together, in a race against Parliament to ensure it’s ready by the time Theresa May triggers Article 50. Don’t ask me to explain why, but it makes me feel slightly better equipped to face the political chaos in which we find ourselves.

And now I have to stifle the feeling of anti-climax as we wait around for her to do the deed at her leisure. I almost feel the impatience of a Brexiteer …

But this isn’t a blog about politics. It’s a blog about knitting, and about the mathematical world that opened itself up to me as I tackled the puzzle of how to position the stars in the EU flag.

Designing the pattern

I found a pattern for individual stars on the wonderful US knitting site, Ravelry.com, but nowhere could I find a pattern where they were arranged in a circle, as in the EU flag. So I had to create one.

The starting point was some basic school trigonometry. There are twelve stars in the flag, arranged in a circle, so the angular distance between each star is 30°. The sine of 30° is 1/2. So, starting from the top, and looking just at the top right-hand quadrant of the circle, the centre of the second star needed to be placed half-way across the knitting.

But how far up/down should it be? This is where it gets messy. The cosine of 30° is √3/2 – an irrational surd. Knitting stitches are discrete, not continuous, so a surd isn’t a great basis for a knitting pattern.

Then I noticed something about √3/2. My calculator told me that the decimal value was very close to 0.866. The difference between 0.866 and 1 is 0.134, which after a few minutes of staring at the calculator started to look a bit more familiar. In fact, 0.134 is pretty close to 2/15, and 0.866 is equally close to 13/15 (of which more below). So as long as I used a multiple of 15 stitches for each quadrant of the circle, I could place my second star to an accuracy that was more than good enough for a knitted cushion: the second star would be 13/15 of the way up from the centre towards the top.

Positioning the third star down was then easy. Here the relevant angle is 60°, and the values of sine and cosine are reversed: sin 60° is √3/2 and cos 60° is 1/2. So, using the approximation above, the third star would be 13/15 of the way across from centre of the circle, and half-way up from the centre.

And that’s it. Similar logic works for each quadrant, giving the position of all 12 stars in the circle.

For knitting purists, there is just one further point I should mention: the thorny question of “tension” (or, in the US, “gauge”). Most knitting stitches are rectangular rather than square: generally they are shorter than they are wide. So in order to form a square grid as the basis for a circle, the knitting needs to have more rows vertically than it has stitches horizontally. This doesn’t affect the fractions in the placement of the stars, because they are based on just one dimension (either rows or stitches) in each case. But it does mean you have to get the right weighting between the numbers of rows and the number of stitches, or you’ll get an ellipse/oval rather than a pure circle.

In the case of my cushion, I used a ratio of approximately 3 rows to 2 stitches in the pattern – though the final result is very slightly adrift of that.

Some mathematical fun

And now for some maths that I hadn’t done at school. [Health warning: if the sines and cosines made your stomach churn, you may want to look away at this point …]

Is it just a coincidence that 13/15 is such a good approximation for √3/2?

No, it isn’t a coincidence, and the reason (or at least one reason) lies in “continued fractions”. An irrational number can be expressed as a continued fraction, and rational approximations can be obtained by cutting off the fraction at an arbitrary depth.

The form of the continued fraction for a square root is highly regular:

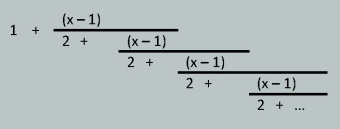
So for √3 we get:

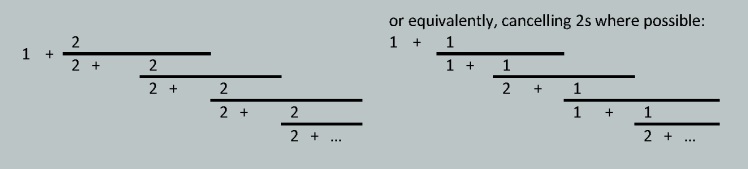
The first step of this approximation, cutting off the fraction after the first number in the denominator, produces the very rough approximation of 2 (1 + 2/2). The next two results, 1⅔ (1 + 2/(2+1)), and 1¾(1 + 2/2⅔), are also pretty wide of the mark. But the next one – 19/11 – is accurate to within 0.5%. The next one again (5 levels deep now) is where 26/15 crops up. This is accurate to within 0.1%, which is sufficient for many practical purposes – as I found in my knitting, where I used 13/15 as a substitute for √3/2.

It’s possible to go arbitrarily deep by continuing the fraction – but the next two results (71/41 and 97/56) are already within 0.02% and 0.01% respectively. The values spiral around √3, alternately higher and lower, and a few steps further on we arrive at Archimedes’ approximations of 265/153 and 1351/780.

But returning to 13/15, there’s another intriguing aspect to explore. As already mentioned, this is an approximation to √3/2. But √3/2 can also be written as √(3/4). And it turns out that the following approximations are also pretty accurate:

17/19 approximates to √(4/5) – or equivalently, 2/√5

21/23 approximates to √(5/6)

25/27 approximates to √(6/7)

and so on …

In fact, it was this series that lured me into the maze of continued fractions in the first place: I started to explore 17/19 out of curiosity, to see if 13/15 was merely a coincidence, and then I realised it was part of a pattern.

So what’s going on here? Again it comes down to continued fractions. If you expand the continued fraction for √((n-1)/n)) using the formula for square roots given above – which I’m not going to do here as it’s fiddly and beyond my ability to format – you’ll find that cutting it off at the second denominator produces the formula (4n + 1)/(4n + 3), giving the series 5/7, 9/11, 13/15, 17/19, 21/23, 25/27 etc. The values with low n are quite poor approximations, but they get better for higher n.

Maths and knitting

The mathematical aspects of knitting are one of the reasons I love it, particularly since I resumed recently after a gap of 30 years. For example, I’ve got plans to knit a blanket in the form of a 10 x 10 “Graeco-Latin square” – a structure that Leonhard Euler, the great 18th-century mathematician, reckoned was impossible, but was proved to exist in 1959.

I like to imagine physicists will eventually discover that the universe is akin to a multi-dimensional piece of knitting – and the recent theory of “loop quantum gravity” suggests that idea might not be wholly fanciful.

But for today I think the lesson is that knitting the EU flag stretches the mind, and is a great distraction from politics.